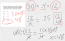Discussion
How many
ounces of water must be added to a 30-ounce solution that is 40 percent alcohol
to dilute the solution to 25 percent alcohol?
*This question is included in Nova Press: Problem Solving Diagnostic Test, question #26
| (A) | 9 |
| (B) | ... |
| (C) | ... |
| (D) | ... |
| (E) | ... |
| (F) | ... |
The solution is
Posted: 11/24/2011 04:06
Please can you provide another explanation to this problem.
It's Q 26/30 of the math d.test
Thanks
It's Q 26/30 of the math d.test
Thanks
Posted: 11/24/2011 13:41
A solution for flamenco boy.
Posted: 11/25/2012 10:48
40/25=1.6
30x1.6=48
By how much 48 is greater than 30, you can find out.
30x1.6=48
By how much 48 is greater than 30, you can find out.
Posted: 12/06/2012 14:30
My approach was to find out how much alcohol was in the original 30-ounce solution
40% of 30 = 12
Since no more alcohol will be added to the new solution, then 12 (the amount of alcohol) must be equal to 25% of the new solution. Therefore the total of the new solution must be
12 divided by .25 or 1/4 = 48
Since we already have 30 ounces, the required additional ounces will be
48-30=18
40% of 30 = 12
Since no more alcohol will be added to the new solution, then 12 (the amount of alcohol) must be equal to 25% of the new solution. Therefore the total of the new solution must be
12 divided by .25 or 1/4 = 48
Since we already have 30 ounces, the required additional ounces will be
48-30=18